Facebook
Google
GitHub
Linkedin
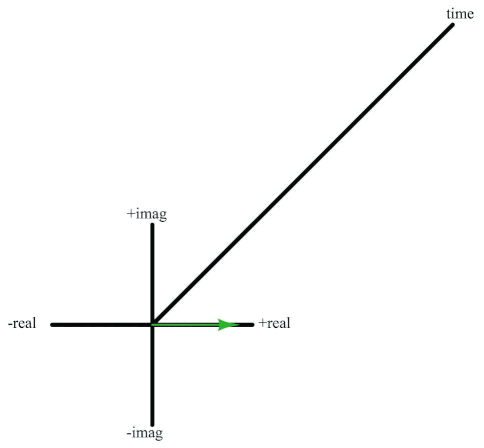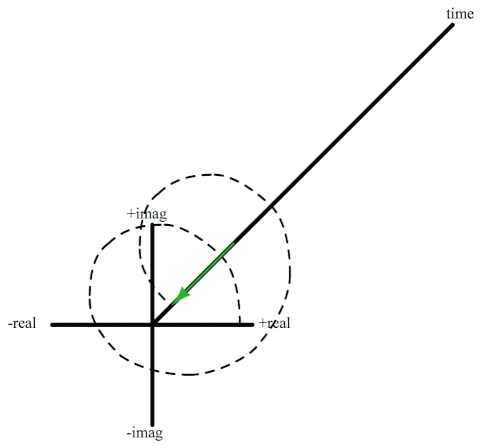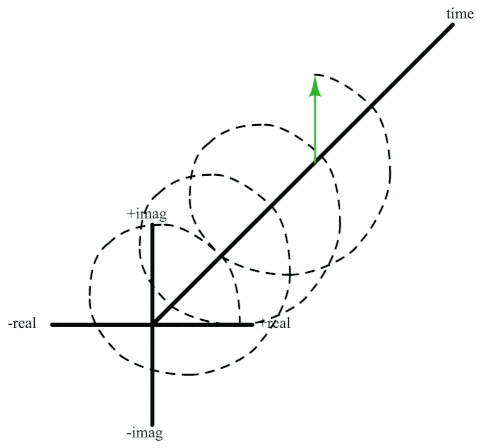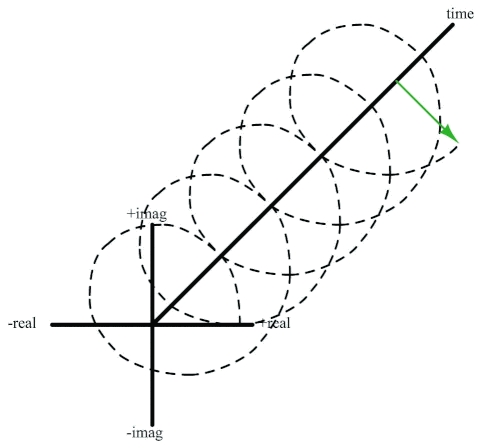
Rotating Phasor in Three-dimensional Form
The following animation shows a rotating phasor in three-dimensional form. The phasor rotates in a complex plane (with real and imaginary axes), but travels linearly along the time axis. In doing so it traces a path that looks like a circle when viewed along the centerline (time axis) but looks like a sinusoidal wave when viewed from above or along the side.
[animation_rotating_phasor]
Euler’s Relation describes the phasor’s position in the complex plane:
\[e^{j \omega t} = \cos \omega t + j \sin \omega t\]
Where,
\(e\) = Euler’s number (approximately equal to 2.718281828)
\(\omega\) = Angular velocity, in radians per second
\(t\) = Time, in seconds
\(\cos \omega t\) = Horizontal projection of phasor (along a real number line) at time \(t\)
\(j \sin \omega t\) = Vertical projection of phasor (along an imaginary number line) at time \(t\)
If you imagine the phasor’s length either growing or decaying exponentially over time, the result will be a spiral that either widens like a horn or shrinks like a funnel. This would be a visualization of a complex exponential, where the \(s\) variable defines both the rate of growth/decay (the envelope of the spiral) and the angular velocity (the pitch of the spiral):
\[e^{st} = e^{(\sigma + j\omega)t} = e^{\sigma t} e^{j \omega t}\]
Where,
\(s\) = Complex growth/decay rate and frequency (sec\(^{-1}\))
\(\sigma\) = \(1 \over \tau\) = Real growth/decay rate (time constants per second, or sec\(^{-1}\))
\(j \omega\) = Imaginary frequency (radians per second, or sec\(^{-1}\))
\(t\) = Time (seconds)
The example of a unit-length rotating phasor is nothing more than a special case of the complex exponential, where \(\sigma = 0\) (i.e. there is no growth or decay over time):
\[e^{st} = e^{(0 + j\omega)t} = e^{0} e^{j \omega t} = e^{j \omega t}\]
